how to find least common multiple

Least Common Multiple

The abbreviation LCM stands for Least Common Multiple. The least common multiple of a number is the smallest number that is the product of two or more numbers. The least common multiple can be calculated for two or more integers as well as two or more fractions. The least common multiple of two numbers is the lowest possible number that can be divisible by both numbers.

There is more than one method to find the LCM of two numbers. One of the quickest ways to find the LCM of two numbers is to use the prime factorization of each number and then the product of the least powers of the common prime factors will be the LCM of those numbers. Explore the world of LCM by going through its various aspects and properties.

1.	What is Least Common Multiple (LCM)?
2.	How to find the Least Common Multiple?
3.	Formulas of LCM
4.	Relationship Between LCM and HCF
5.	Difference Between LCM and HCF
6.	Properties of LCM
7.	FAQs on LCM

What is Least Common Multiple (LCM)?

The least common multiple is also known as LCM (or) the Lowest Common Multiple in Math. The least common multiple of two or more numbers is the smallest number among all common multiples of the given numbers. Let's take two numbers: say, 2 and 5. Each will have its own set of multiples.

Multiples of 2 are 2, 4, 6, 8, 10, 12, 14, 16, 18, 20, …
Multiples of 5 are 5, 10, 15, 20, …

Let's represent these multiples on the number line and circle the common multiples,

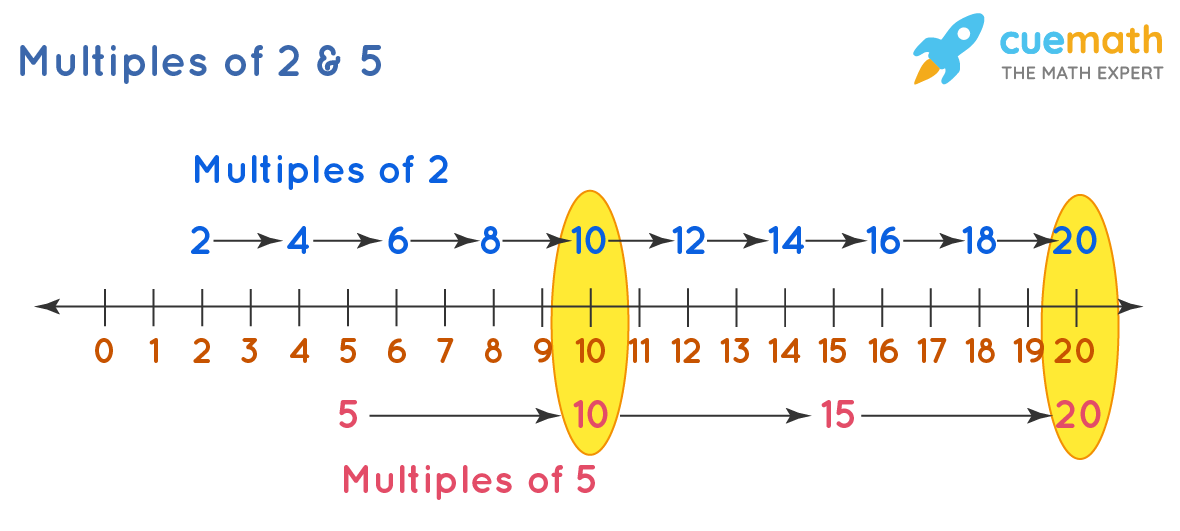
Least Common Multiples of 2 and 5

Thus, the common multiples of 2 and 5 are 10, 20, ….. The smallest number among 10, 20, … is 10. So the least common multiple of 2 and 5 is 10. Therefore,LCM (2, 5) = 10

How to find the Least Common Multiple?

LCM of numbers can be calculated using various methods. There are 3 methods to find the least common multiple of two numbers. Each method is explained below with some examples of LCM.

LCM by Listing Method
LCM using Prime Factorization
LCM using Division Method

LCM by Listing Method (Listing Out the Common Multiples)

By using the listing out the common multiples method we can find out the common multiples of two or more numbers. Out of these common multiples, the least common multiple is considered and the LCM of two given numbers can thus be calculated. To calculate the LCM of the two numbers A and B by using listing out the common multiples follow the steps given below:

Step 1 - List a few multiples of A and B
Step 2 - Mark the common multiples from the multiples of both numbers.
Step 3 - Select the smallest common multiple, that smallest common multiple is the LCM of the two numbers.

Example: Find the least common multiple (LCM) of 4 and 5.

Solution: Multiples of 4 are: 4, 8, 12, 16, 20, 24, 28, 32, 36, 40, ... and the multiples of 5 are: 5, 10, 15, 20, 25, 30, 35, 40, ...

LCM of 4 and 5 by listing out common multiples

Hence, by the definition of least common multiple, the LCM of 4 and 5 is 20

LCM using Prime Factorization

By using the prime factorization method we can find out the prime factors of the numbers and these prime factors can be used to find the LCM of those numbers. To calculate the LCM of two numbers using the prime factorization method follow the steps given below:

Step 1 - Represent the numbers in the prime factored form.
Step 2 - The LCM of the given two numbers is the product of all the prime factors. (However, common factors will be included only once)

Let's learn this method using the example given below.

Example: Find the least common multiple (LCM) of 60 and 90 usingprime factorization.

Solution:

Step 1 - The prime factorization of 60 and 90 are: 60 = 2 × 2 × 3 × 5 and 90 = 2 × 3 × 3 × 5
Step 2 - The product of all the prime factors = 2 × 2 × 3 × 5 × 3 = 180.

LCM of 60 and 90 by Prime Factorization

Therefore, LCM of 60 and 90 = 2 × 2 × 3 × 5 × 3 = 180

By Division Method

By the division method, we will divide the numbers by a common prime number, and these dividends are used to calculate the LCM of those numbers. To calculate the LCM of two numbers using the division method follow the steps given below:

Step 1 - Find a prime number which is a factor of at least one of the given numbers. Write this prime number on the left of the two numbers. For each number in the right column, do the following:
Step 2 - If the prime number in step 1 is a factor of the number, then divide the number by the prime and write the quotient below. If the prime number in step 1 is not a factor of the number, then write the number in the row below as it is. Continue the steps until all coprime numbers are left in the last row. (Two numbers are said to be coprime if they share no common factors other than 1.)

Let's learn this method using the example given below.

Example: Find the least common multiple (LCM) of 6 and 15 using the common division method.

Solution:

Step 1 - 2 is the smallestprime number and itis a factor of 6. Write this prime number on the left of the two numbers. For each number in the right column, continue finding out prime numbers which are their factors.
Step 2 - 2 divides 6 but it's not a factor of 15, then write the number 15 in the row below as it is. Continue the steps until all coprime numbers are left in the last row.
Step 3 - The LCM is the product of all the prime numbers. LCM of 6 and 15 is, 2 × 3 × 5 = 30

Though we have three methods to find the LCM, the prime factorization method is the most common and easy method that we use.

Formulas of LCM

LCM formulas are the collection of the numbers their LCM, HCF(Highest Common Factor), and their GCD(Greatest Common Divisor). These formulas are used to calculate the least common multiple of two integers as well as the LCM of two fractions. The LCM formulas for integers and fractions are shown below.

LCM Formula for Integers

If a and b are the two integers then the formula for their least common multiple is given as:

LCM (a,b) = (a x b)/GCD(a,b)

LCM Formula for Fractions

If a/b and c/d are the two fractions then the formula for their least common multiple is given as:

LCM (a/b, c/d) = (LCM of Numerators)/(HCF of Denominators) = LCM (a,c)/HCF (b,d)

Relationship Between LCM and HCF

HCF of two or more numbers is the highest common factor of the given numbers. It is calculated by multiplying the common prime factors of the given numbers. Whereas the least common multiple of two or more numbers is the smallest number among all common multiples of the given numbers.
Let's assume a and b are the two numbers then the formula that gives the relationship between their LCM and HCF is given as:

LCM (a,b) × HCF (a,b) = a × b

Difference Between LCM and HCF

The HCF or the Highest common factor of two or more numbers is the highest or the greatest factor among all the common factors of the given numbers, whereas the LCM or the least common multiple of two or more numbers is the smallest number among all common multiples of the given numbers. The following table shows the difference between HCF and LCM:

LCM (Lowest Common Multiple)	HCF (Highest Common Factor)
The least common multiple of two or more numbers is the smallest number among all common multiples of the given numbers.	The highest common factor of two or more numbers is the highest number among all the common factors of the given numbers.
The respective numbers are the factors of their LCM.	HCF of two or more numbers is a factor of each of the numbers.
LCM of two or more prime numbers can never be 1.	HCF of two or more prime numbers is 1 always.
LCM of two or more numbers is always greater than or equal to each of the numbers.	HCF of two or more numbers is always less than or equal to each of the numbers.

Properties of LCM

The least common multiple of two or more numbers is having a wide number of properties. Given below are the three most common properties of the LCM:

Associative Property: This property is applicable for two numbers:LCM (a,b) = LCM (b,a)
Commutative Property: This property is only applicable for three numbers:LCM (a,b,c) = LCM (a, LCM (b,c)) = LCM (LCM (a,b),c)
Distributive property: This property is only applicable for four numbers:LCM (da, db, dc) = d × LCM (a, b, c)

Example 1: Find the LCM of 980 and 9000 using the prime factorization method.

Solution:

First, we carry out the prime factorization of the two numbers: 980 = 2 × 2 × 5 × 7 × 7 = 2² × 5¹ × 7² and 9000 = 2 × 2 × 2 × 3 × 3 × 5 × 5 × 5 = 2³ × 3² × 5³. The LCM will be the product of the greatest power of each prime factor occurring in the numbers: 2³ × 3² × 5³ × 7² = 441000

∴ LCM (980, 9000) = 2³ × 3² × 5³ × 7² = 441000
Example 2:Bus X comes to a stop every 15 minutes and bus Y comes to the same stop every 40 minutes. Let us assume that both buses are currently at the same bus stop. After how many hours will both the buses reach the same stop again?

Solution:

To find the required time, we have to find the LCM of 15 and 40. The multiples of 15 are 15, 30, 45, 60, 75, 90, 105, 120, 135, 150.... and the multiples of 40 are 40, 80, 120, 160,... So the LCM of 15 and 40 is 120. Thus, both buses reach the same stop again after 120 minutes, which is the same as 2 hours.

∴ Required time = 2 hours

go to slidego to slide

Have questions on basic mathematical concepts?

Become a problem-solving champ using logic, not rules. Learn the why behind math with our certified experts

Book a Free Trial Class

FAQs on Least Common Multiple (LCM)

How do I Find the Least Common Multiple in Math?

There are 3 methods to calculate the LCM of two numbers: by listing out the common multiples, by prime factorization method, and by division method. These methods have been explained in the previous sections with examples.

What is the Fastest and Easy Way to Find the LCM?

Though we have three methods to find the LCM, listing out the common multiples, prime factorization method, and division method. Out of these three methods, the prime factorization method is the most common, fastest, and easiest method.

What is the LCM of 12 and 9?

First few multiples of 12 are 12, 24, 36, 48, 60, 72, 84, ... and first few multiples of 9 are 9, 18, 27, 36, 45, … The LCM of 12 and 9 is the smallest multiple that is a common multiple of both of the numbers. Thus, the LCM of 12 and 9 is 36.

What is the Difference Between LCM and HCF?

The least common multiple of two or more numbers is the smallest number among all common multiples of the given numbers and the HCF (Highest Common Factor) of two or more numbers is the highest number among all the common factors of the given numbers.

What is the Relationship Between HCF and LCM of Two Numbers?

Let's assume a and b are the two numbers. Then the formula that gives the relationship between their LCM and HCF is given as; LCM (a,b) × HCF (a,b) = a × b. The LCM of 12 and 8 is the smallest number among all common multiples of both of the numbers. Thus, the LCM of 12 and 8 is 24.

What are the Properties of LCM?

The three most common properties of the LCM are given below:

Associative Property: This property is applicable for two numbers: LCM (a,b) = LCM (b,a)
Commutative Property: This property is only applicable for three numbers: LCM (a,b,c) = LCM (a, LCM (b,c)) = LCM (LCM (a,b),c)
Distributive property: This property is only applicable for four numbers: LCM (da, db, dc) = d × LCM (a, b, c)